Universality in Biology

Biology: Amyloid fibrillisation | Physics: Living polymeric systems
Amyloid fibrils are fibrous aggregates of proteins (Fig. 2A). Their significance is profound: they are implicated in devastating human diseases such as Alzheimer’s, Parkinson’s, and type II diabetes. Over the past decade, my work has developed a theoretical framework for amyloid fibrilisation. This includes investigations into the phase behaviour of amyloid-forming proteins [1]; the universal kinetics of fibrilisation in the high bonding energy limit [2]; the central role of interfacial effects in fibril formation [3–6]; and the interplay between gelation and phase separation during amyloidogenesis in vitro [7,8]. Many of these studies emerged from close collaboration with the Vaux group at the Dunn School of Pathology in Oxford.

These insights now set the stage for the next frontier: amyloid pathogenesis in vivo. In living systems, fibrilisation unfolds under fundamentally different conditions — proteins are continuously produced, degraded, and modified, placing the system in a far-from-equilibrium regime. To bridge the in vitro–in vivo gap, we must therefore treat amyloid fibrilisation as a problem in the physics of “living” polymeric systems. Understanding this regime will be key to explaining how amyloid diseases actually arise within the body.

Biology: Intracellular organisation via phase separation | Physics: Non-equilibrium phase separation
Cells depend on intricate organisation to function properly. While some organelles are membrane-bound, many are not. Since 2009 [9], it has become clear that non-membrane-bound organelles form via phase separation (Fig. 2B). This realisation has transformed our understanding of cell biology and is rewriting the textbooks.

The theoretical framework for equilibrium phase separation is well established. Yet, cells are inherently non-equilibrium systems, continually driven by ATP hydrolysis and other energy-consuming processes. In such contexts, equilibrium theories become inadequate. One striking example is that the classical Lifshitz-Slyozov scaling law [10], which describes coarsening dynamics in equilibrium systems, can break down in driven phase-separating systems [11,12].

I have been fortunate to help shape this emerging field, contributing to fundamental theoretical advances [12–15] and co-authoring two recent reviews [16,17]. Nevertheless, the field still lacks a comprehensive theory of non-equilibrium phase separation that is both physically rigorous and biologically relevant. Formulating such a theory, and connecting it directly to cellular function, is a central aim of my group.

Biology: Active cell, tissue, and organism dynamics | Physics: Active matter
Life is motion. From the migration of individual cells to the coordinated flow of tissues, from wound healing to bacterial colony expansion, motility defines living systems (Fig. 2C). Understanding these active processes is essential to explaining how organisms develop, heal, and adapt.

Beyond biology, the physics of active matter has emerged as a rich source of discovery, revealing new transitions, phases, and collective phenomena. In the past five years, I have co-discovered two new universality classes using analytical renormalisation group approaches: one describing a novel critical phenomenon in incompressible active fluids [18], and another identifying a fundamentally new non-equilibrium state of matter [19]. Building a classification of active matter universality classes — akin to the periodic table for chemical elements — is a major ongoing ambition.

At the same time, my group applies active matter theory back to biological systems, closing the conceptual loop highlighted in Fig. 1. For example, in collaboration with Prof. Darryl Overby (Imperial College), we are exploring how the active dynamics of the cell cortex influence intracellular pore formation in glaucoma. With Prof. Cristina Lo Celso (Imperial College), we are studying stem cell regeneration in bone marrow through the lens of motility-induced phase separation. These projects exemplify how theoretical physics and biology can be woven together to generate new understanding on both sides.

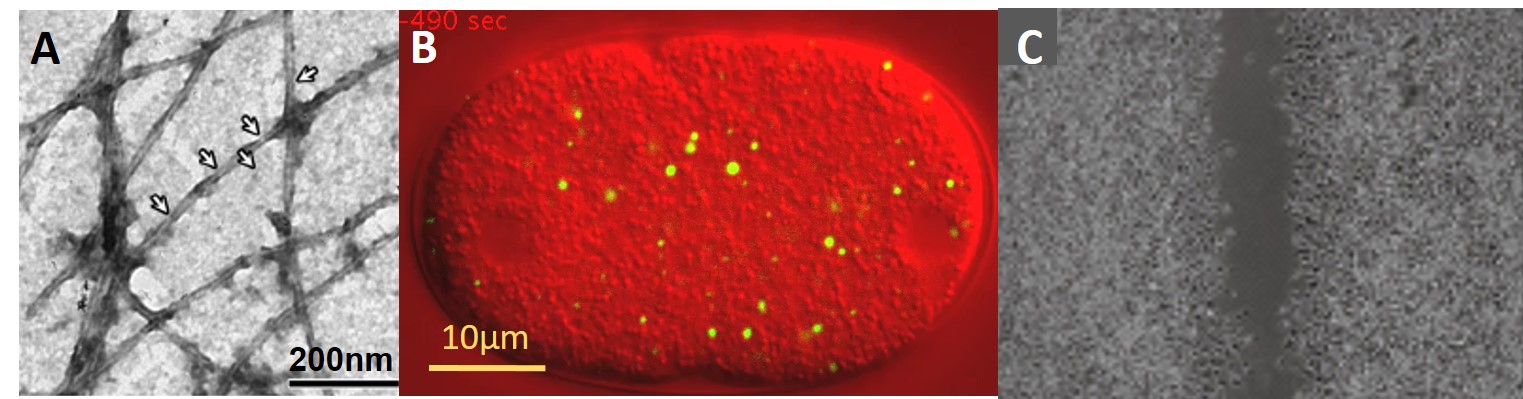
Figure 2: (A) Biopolymer self-assembly. Amyloid fibrils formed from Aβ proteins implicated in Alzheimer’s disease [5]. (B) Non-equilibrium phase separation in C. elegans embryos [9,13]. (C) Wound healing assay viewed as an active matter system, whose large-scale behaviour is described by the Toner–Tu equations, just as the Navier–Stokes equations describe passive fluids.

Vision
Across these diverse problems, three themes recur: universality, non-equilibrium dynamics, and phase transitions. These form the foundation of my research group, Universality in Biology. Our mission is to uncover the universal behaviours that govern biological systems operating far from equilibrium.

The significance of this endeavour spans disciplines. For physicists, biology offers an arena where new universality classes and non-equilibrium principles can be uncovered. For biologists, universality offers a unifying language: a way to connect systems that appear unrelated, to make predictions that are robust to microscopic details, and to explain emergent, system-level properties. I anticipate that in the near future, the concept of universality will become part of every biologist’s toolkit, and researchers will identify the universality class to which their system belongs.

The broader impact reaches into materials science and bioengineering. Advances in our ability to design and manipulate living matter — from microbial cell factories to engineered tissues — mirror the revolution brought by semiconductor materials in the mid-20th century. Just as semiconductors propelled condensed matter physics to prominence in the 1960s [20], I believe that biological physics will become the next transformative frontier.

By pursuing universality in biology, we not only deepen our understanding of life itself but also lay the groundwork for a new physics of living matter — one that will drive both scientific discovery and technological innovation.